What is Topology? A Complete Overview

Topology, a branch of mathematics, explores the properties of space that remain unchanged under continuous deformations such as stretching, crumpling, and bending. It is a fundamental field that has applications not only in mathematics but also in various scientific disciplines and real-world problems. In this comprehensive overview, we will delve into the fundamentals of topology, its key concepts, and its wide-ranging applications.

Whether you’re a seasoned mathematician or someone just starting to explore the world of numbers, pi123 caters to all skill levels. All Information about Pi123 is that its versatility ensures that it remains a valuable resource for beginners and experts alike, fostering a love for mathematics in users of every background.

1. Introduction

Defining Topology

Topology is a branch of mathematics that investigates the properties of space that are preserved under continuous deformations. Unlike geometry, which is concerned with distances and angles, topology focuses on the broader concept of spatial relationships that remain invariant under transformations. Topology seeks to understand the fundamental nature of shapes and spaces, classifying them based on their inherent properties.

Historical Development

The origins of topology can be traced back to the 18th century, but the field truly began to take shape in the early 20th century with the groundbreaking work of mathematicians like Henri Poincaré and Felix Hausdorff. Poincaré’s work on homology and the concept of a fundamental group laid the foundation for algebraic topology, while Hausdorff’s investigations into topological spaces and the separation axioms established key concepts in point-set topology.

2. Basic Concepts of Topology

Topological Spaces

At the heart of topology is the notion of a topological space. A topological space is a set equipped with a collection of open sets that satisfy certain properties. These properties capture the essence of continuity and neighborhood relations, allowing for a flexible and abstract approach to studying spatial structures.

Open and Closed Sets

Open sets are a fundamental concept in topology, representing sets in which every point has a neighborhood entirely contained within the set. Closed sets are complements of open sets. The interplay between open and closed sets forms the basis for defining various topological structures.

Continuity and Homeomorphisms

Topology provides a generalized notion of continuity. A function between two topological spaces is considered continuous if the inverse image of every open set is open. Homeomorphisms are bijections between spaces that preserve the topological structure, indicating that the spaces are essentially the same from a topological perspective.

3. Topological Structures

Basis and Subbasis

In defining a topology, one can use a basis or a subbasis. A basis is a collection of open sets from which all other open sets can be generated. A subbasis is a smaller collection that generates the basis. These concepts provide alternative approaches to constructing topologies.

Topological Manifolds

Topological manifolds are spaces that locally resemble Euclidean spaces. They are important in various branches of mathematics and physics. Differentiable manifolds, which introduce a smooth structure, extend the concept to spaces that allow calculus-like operations.

Metric Spaces

While topology is concerned with abstract spatial relationships, metric spaces bring a quantitative aspect to the study of spaces by incorporating a distance function. Metric spaces provide a bridge between topology and more concrete geometric concepts.

4. Fundamental Concepts in Topology

Connectedness

Connectedness is a key topological property that characterizes the extent to which a space is in one piece. A space is connected if it cannot be divided into two disjoint non-empty open sets. The study of connectedness helps classify and understand the structure of spaces.

Compactness

Compactness is a generalization of boundedness. A space is compact if, intuitively, it is not “too large” and “not too spread out.” Compact spaces have important properties that make them amenable to analysis and applications in areas like functional analysis and optimization.

Convergence and Limit Points

Convergence in topology is a generalization of the concept from analysis. A sequence of points in a space converges to a limit if, intuitively, the points get arbitrarily close to the limit. Limit points play a crucial role in defining continuity and understanding the structure of spaces.

5. Algebraic Topology

Homotopy and Homotopy Equivalence

Homotopy is a fundamental concept in algebraic topology that captures the idea of continuous deformations between maps. Spaces that are homotopy equivalent share certain topological properties, leading to a broader classification of spaces.

Fundamental Group

The fundamental group is a topological invariant that associates algebraic structures with topological spaces. It measures the number of “holes” in a space and provides a powerful tool for distinguishing between different spaces. The concept has applications in fields such as knot theory and robotics.

Homology and Cohomology

Homology and cohomology are algebraic invariants that associate algebraic structures (groups) to topological spaces. They provide a means of distinguishing spaces and offer insights into their “shape.” These concepts have applications in a wide range of areas, including differential geometry and physics.

6. Applications of Topology

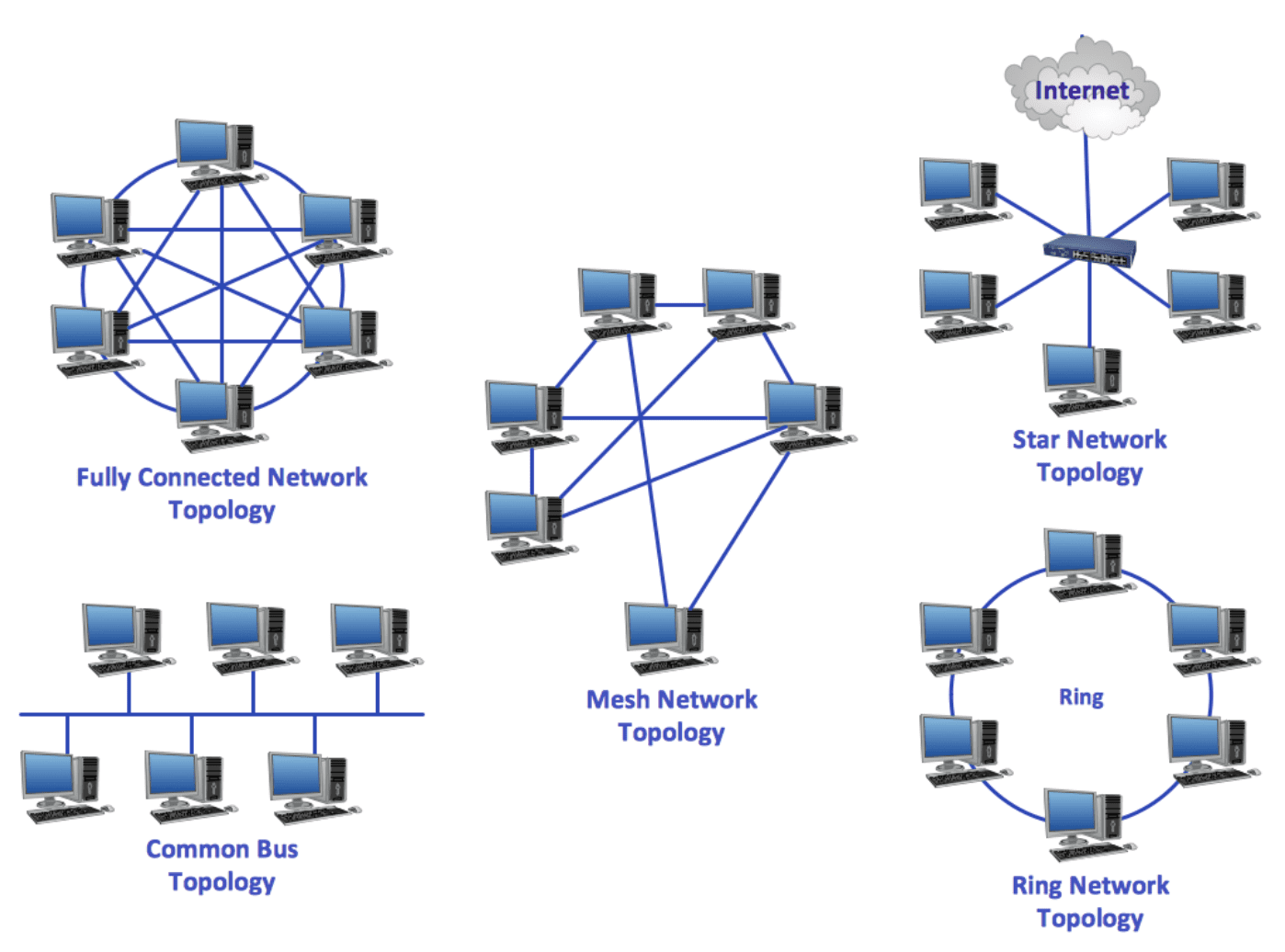
Network Topology

Topology is widely used in the field of computer science, particularly in the design and analysis of computer networks. Network topology studies the arrangement of elements (links, nodes) in a network, helping optimize communication efficiency and fault tolerance.

GIS (Geographic Information Systems)

Geography and spatial analysis benefit from topology in Geographic Information Systems (GIS). Topological relationships between geographic features, such as adjacency and connectivity, are crucial for accurate spatial modeling and analysis.

Robotics and Motion Planning

In robotics, topology plays a role in motion planning and sensor networks. Understanding the topological properties of the environment allows robots to plan efficient paths and navigate complex spaces.

7. Advanced Topics in Topology

Topological Groups

Topological groups merge the concepts of group theory and topology. A topological group is a group equipped with a topology such that the group operations are continuous. These structures have applications in areas like Lie theory and harmonic analysis.

Knot Theory

Knot theory is a branch of topology that studies the mathematical properties of knots. Knots are closed loops embedded in three-dimensional space. The study of knots has connections to physics, biology, and chemistry.

Topological Quantum Field Theory

In theoretical physics, topological quantum field theory explores the relationship between topology and quantum field theory. These theories provide a framework for understanding certain aspects of quantum mechanics and quantum computing.

8. Challenges and Open Problems

Poincaré Conjecture

The Poincaré Conjecture, posed by Henri Poincaré in the early 20th century, was one of the most famous open problems in topology. It was eventually proven by Grigori Perelman in 2003, marking a significant milestone in the field.

Four Color Theorem

The Four Color Theorem, initially conjectured in the 19th century, states that every planar map can be colored with four colors in such a way that no two adjacent regions have the same color. The theorem was proven with the aid of computers in the 20th century.

Millennium Prize Problems

Several other open problems in topology and related areas are part of the Millennium Prize Problems, including the Hodge Conjecture, Navier-Stokes Existence and Smoothness, and the Birch and Swinnerton-Dyer Conjecture.

9. Future Directions and Emerging Trends

Topological Data Analysis

Topological Data Analysis (TDA) is an emerging field that applies topological methods to analyze complex datasets. TDA provides a unique perspective on the shape and structure of data, finding applications in fields such as machine learning and biology.

Quantum Topology

The intersection of topology and quantum physics is leading to the development of quantum topology. This emerging field explores the topological aspects of quantum systems and their potential applications in quantum computing.

Applications in Machine Learning

Topology is finding applications in machine learning, particularly through techniques like persistent homology. These methods enable the extraction of topological features from data, enhancing the understanding of complex relationships.

Conclusion

Topology, with its abstract and versatile approach to spatial relationships, has become a cornerstone in mathematics and beyond. From the foundational concepts of topological spaces and continuity to advanced topics like algebraic topology and applications in diverse fields, topology continues to evolve and shape our understanding of the world around us. As researchers tackle open problems, explore emerging trends, and apply topological methods to new frontiers, the field remains a vibrant and dynamic force in the landscape of mathematical exploration.